Banan Kerdi, Mathieu Pierre, Michel Goiran, and Walter Escoffier, LNCMI Toulouse.
Transition-metal dichalcogenides (TMDCs) are two-dimensional layered materials showing intriguing electronic properties, especially when thinned down to a single layer. We studied WSe2 monolayers fabricated at LNCMI-Toulouse through transport measurements.
We measured the magnetoresistance at 4.2 K under pulsed magnetic fields up to 55 T for several hole densities. We attribute the complex Shubnikov-de Haas oscillations to a partially resolved, large Zeeman splitting of the valence band in this material, thanks to the presence of heavy tungsten atoms as well as electronic interactions.
We developed a model to simulate the magnetoresistance oscillations, in which the main parameter is the ratio between the Zeeman energy Ez and the cyclotron energy Eс. It takes into account the Landau levels (LLs) from both valleys (i.e., |K ↑> and |K’ ↓> states, due to spin-valley locking) with the same Gaussian broadening.
Although a shift of ±2 of Ez/Ec gives the same apparent splitting between the |K ↑> and |K’ ↓> LLs, we could determine the value of this ratio by studying (i) the relative amplitude of the resistance oscillations, corresponding to the crossing of the chemical potential with the LLs, which depends on their index; (ii) the transition from a mixed to a polarized LL regime which takes place at a critical field depending on Ez/Ec. We found that the Zeeman energy is several times larger than the cyclotron energy.
Our model relies on the Landau-level spectrum of the charge carriers. In TMDC monolayers, the nature of the charge carriers as Schrödinger fermions (SCHFs) or massive Dirac fermions (MDFs) remains controversial. Regarding their LL spectrum, the main difference lies in the double degeneracy of the zeroth Landau level. The choice of the fermion model translates into a shift of 1 of Ez/Ec.
The effective Landé factor of the holes is computed from g* = 2 × me/m* × Ez/Ec. In the explored range of hole densities (between 7.5 and 5 × 1012 cm-2), this factor ranges between 14.7 and 17.3 for MDFs and between 19.1 and 21.8 for SCHFs, considering a constant effective mass (m* = 0.45 × me) of the holes. g* follows the trend previously reported in the literature and is found to increase when the hole density is reduced, confirming the importance of electronic interactions in understanding the electronic properties of this material.
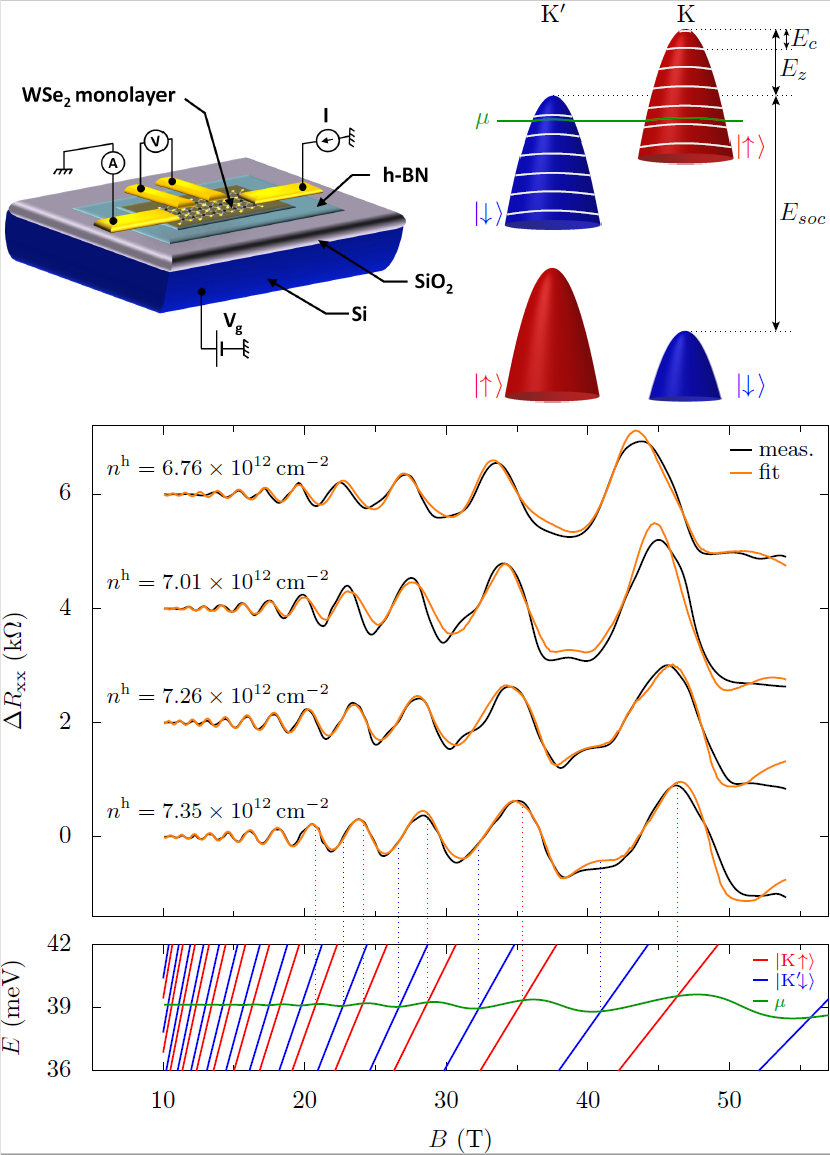
Figure: (Top) Sketch of the WSe2 monolayer sample and simplified structure of the valence band in a magnetic field. (Middle) High-field magnetoresistance of the sample at T = 4.2 K, after background removal, for various values of the hole density. The fit obtained with our model is superimposed. The complex oscillation pattern appearing at high magnetic field due to a non-integer Ez/Ec ratio is well reproduced. (Bottom) Spectrum of the Landau levels of both spin/valley indices and simulated evolution of the chemical potential µ(B).
High magnetic field spin-valley-split Shubnikov–de Haas oscillations in a WSe2 monolayer, B. Kerdi, M. Pierre, R. Cours, B. Warot-Fonrose, M. Goiran, and W. Escoffier, Phys. Rev. B 102, 155106 (2020). https://journals.aps.org/prb/abstract/10.1103/PhysRevB.102.155106
Contact: mathieu.pierre@lncmi.cnrs.fr walter.escoffier@lncmi.cnrs.fr